Classical painting techniques in Renaissance art

Classical painting techniques: Perspective

Part 3

The use of Perspective: The first major treatment of the painting as a window into space appeared in the work of Giotto di Bondone, at the beginning of the 14th century. True linear perspective was formalized later, by Filippo BrunelleschiLeon Battista Alberti. In addition to giving a more realistic presentation of art, it moved Renaissance painters into composing more paintings.

Pietro Perugino's usage of perspective in this fresco at the Sistine Chapel (1481–82) helped bring the Renaissance to Rome.

Prior to the Renaissance, a clearly modern optical basis of perspective was given in 1021, when Alhazen (al-Hasan Ibn al-Haytham, d. ca. 1041 CE), an Iraqi physicist and mathematician, in his Book of Optics (Kitab al-manazir; known in Latin as De aspectibus or Perspectiva), explained that light projects conically into the eye. Alhazen’s geometrical, physical, physio-psychological optics resolved in this the ancient dispute between the mathematicians (Ptolemaic and Euclidean) and the physicists (Aristotelian) over the nature of vision and light. He also showed that vision is not merely a phenomenon of pure sensation (namely what results from the introduction of light rays into the eyes), but that essentially it involves the faculties of judgement, imagination and memory. Alhazen’s geometrical model of the cone of vision was theoretically sufficient to translate visible objects within a given setting into a painting, and this was also supported by his experimental affirmation of the visibility of spatial depth; hence of offering a proper ground for the idea of perspective. Moreover, Alhazen presented a geometrical conception of place as spatial extension (a postulated void), and he refuted the Aristotelian account of topos as a surface of containment. Alhazen’s mathematical definition of place was more akin to Plato’s notion of Khôra or Chora as ‘space’, yet conceived on pure geometric grounds to facilitate the use of projections. In all of this, Alhazen was concerned with optics, with vision, light and the nature of colour, as well as with experimentation and the use of optical instruments, and not with painting as such. Conical translations are mathematically difficult, so a drawing constructed using them would be incredibly time consuming. However, what Alhazen named a cone of vision (makhrut al-shu’a’) corresponded also with the idea of a pyramid of vision, hence, offering a model that can be more easily projected in orthogonal drawings of side views and top views that are needed in the geometric construction of perspective.

By the 14th century, Alhazen’s Book of Optics was available in Italian translation, entitled Deli Aspecti. The Renaissance artist Lorenzo Ghiberti relied heavily upon this work, quoting it “verbatim and at length” while framing his account of art and its aesthetic imperatives in the “Commentario terzo.” Alhazen’s work was thus “central to the development of Ghiberti’s thought about art and visual aesthetics” and “may well have been central to the development of artificial perspective in early Renaissance Italian painting.”

The Florence Baptistery or Battistero di San Giovanni

In about 1413 a contemporary of Ghiberti, Filippo Brunelleschi, demonstrated the geometrical method of perspective, used today by artists, by painting the outlines of various Florentine buildings onto a mirror. When the building’s outline was continued, he noticed that all of the lines converged on the horizon line. According to Vasari, he then set up a demonstration of his painting of the Baptistry in the incomplete doorway of the Duomo. He had the viewer look through a small hole on the back of the painting, facing the Baptistry. He would then set up a mirror, facing the viewer, which reflected his painting. To the viewer, the painting of the Baptistry and the Baptistry itself were nearly indistinguishable.

Soon after, nearly every artist in Florence and in Italy used geometrical perspective in their paintings, notably Melozzo da Forlì and Donatello. Melozzo first used the perspective from down to up (in Rome, Loreto, Forlì and others), and was celebrated for that. Donatello started sculpting elaborate checkerboard floors into the simple manger portrayed in the birth of Christ. Although hardly historically accurate, these checkerboard floors obeyed the primary laws of geometrical perspective: all lines converged to a vanishing point, and the rate at which the horizontal lines receded into the distance was graphically determined. This became an integral part of Quattrocento art. Not only was perspective a way of showing depth, it was also a new method of composing a painting. Paintings began to show a single, unified scene, rather than a combination of several.

As shown by the quick proliferation of accurate perspective paintings in Florence, Brunelleschi likely understood (with help from his friend the mathematician Toscanelli), but did not publish, the mathematics behind perspective. Decades later, his friend Leon Battista Alberti wrote De pictura (1435/1436), a treatise on proper methods of showing distance in painting. Alberti’s primary breakthrough was not to show the mathematics in terms of conical projections, as it actually appears to the eye. Instead, he formulated the theory based on planar projections, or how the rays of light, passing from the viewer’s eye to the landscape, would strike the picture plane (the painting). He was then able to calculate the apparent height of a distant object using two similar triangles. The mathematics behind similar triangles is relatively simple, having been long ago formulated by Euclid. In viewing a wall, for instance, the first triangle has a vertex at the user’s eye, and vertices at the top and bottom of the wall. The bottom of this triangle is the distance from the viewer to the wall. The second, similar triangle, has a point at the viewer’s eye, and has a length equal to the viewer’s eye from the painting. The height of the second triangle can then be determined through a simple ratio, as proven by Euclid. Alberti was also trained in the science of optics through the school of Padua and under the influence of Biagio Pelacani da Parma who studied Alhazen’s Optics (see what was noted above in this regard with respect to Ghiberti).

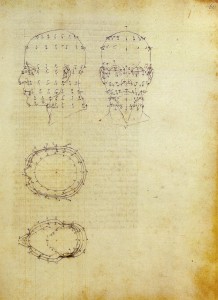
Piero della Francesca elaborated on Della Pittura in his De Prospectiva Pingendi in the 1470s.

Piero della Francesca elaborated on Della Pittura in his De Prospectiva Pingendi in the 1470s. Alberti had limited himself to figures on the ground plane and giving an overall basis for perspective. Della Francesca fleshed it out, explicitly covering solids in any area of the picture plane. Della Francesca also started the now common practice of using illustrated figures to explain the mathematical concepts, making his treatise easier to understand than Alberti’s. Della Francesca was also the first to accurately draw the Platonic solids as they would appear in perspective.

Arnolfini Portrait by Jan van Eyck (London, National Gallery)

Perspective remained, for a while, the domain of Florence. Jan van Eyck, among others, was unable to create a consistent structure for the converging lines in paintings, as in London’s The Arnolfini Portrait, because he was unaware of the theoretical breakthrough just then occurring in Italy. However he achieved very subtle effects by manipulations of scale in his interiors. Gradually, and partly through the movement of academies of the arts, the Italian techniques became part of the training of artists across Europe, and later other parts of the world.